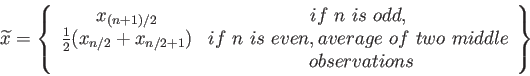Measures of Location: Sample Mean and Median

• Location measures in a data set provide the analyst some quantitative measure of where the data center is in a sample.
• One obvious measure is the sample mean (Mathematical Average), which is simply a numerical average.
• The sample median is to reflect the central tendency of the sample and is uninfluenced by extreme values.
• Suppose that the observations in a sample are $x_1,x_2,\ldots,x_n$. The sample mean, denoted by $\bar{x}$ is
  $$\bar{x}=\sum_{i=1}^n\frac{x_i}{n}=\frac{x_1+x_2+\ldots+x_n}{n}$$
  Sensitive to outliers (or extreme values).
• Sample Median - middle value in the observations of ordered data set. It divides a data set into two equal parts, denoted by $\widetilde{x}$;
  $$\widetilde{x}=\left\lbrace \begin{array}{cc} x_{(n+1)/2} & ... ... two middle\\ &  observations \\ \end{array}\right\rbrace$$

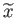• For example, if the data set is the following: 1.7, 2.2, 3.11, 3.9, and 14.7. The sample mean is 5.12 and the sample median is 3.9.
• The centroid of the data

  Figure 1.6: Sample mean as a centroid of the ``with nitrogen'' stem weight.

  

• A trimmed mean is computed by ``trimming away'' a certain percent of both the largest and smallest set of values.
• Example:
  • The 10% trimmed mean is found by eliminating the largest 10% and smallest 10% and computing the average of the remaining values.
  • So, for the with nitrogen group the 10% trimmed mean is

Table 1.3: Observation of nitrogen influences.

No nitrogen	Nitrogen
0.32	0.26
0.53	0.43
0.28	0.47
0.37	0.49
0.47	0.52
0.43	0.75
0.36	0.79
0.42	0.86
0.38	0.62
0.43	0.46

$$\bar{x}_{tr(10)}=\frac{0.43+0.47+0.49+0.52+0.75+0.79+0.62+0.46}{8}=0.56625$$